
Back home
Fench version at: http://jacques-andre.fr/faqtypo/truchet/truchet.html
The full English version is available as a TUGBOAT
paper (pdf 368 Ko)
Truchet The Point Romain du roi Tiling systems

Sébastien Truchet, 1657-1729.
Recall of the previous episode: father Sébastien Truchet, in the Bignon Commission, invented the typographic point and defined the Romain du Roi typeface.
However, at the beginning of the 1700s, Truchet returned to his main field of specialisation: hydraulics. He worked on the Orleans Canal project and noticed (sic) "in a chateau called Motte S. Lyé, 4 leagues just before the town of Orleans several quarried & divided into two colours by a diagonal line, which were intended to cover a chapel and several other apartments". Truchet then looked for a way to combine these tiles to produce agreeable designs and in 1704 he published the findings of his research in Comptes-rendus de l'Académie des Sciences. This concept of tiling was not new but Truchet was to the first to publish a detailed study of all combinations of a tiling system.
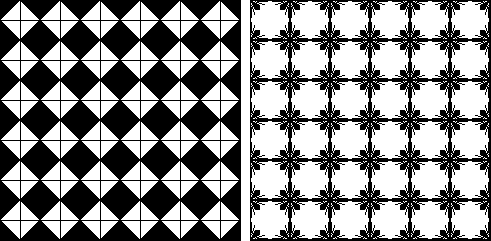
Truchet Pavage (left) and a new design using ornaments à la Fournier (right)
His concept has today become commonplace: if you look closely you will recognise his designs in bathrooms, on kitchen slab floors, in certain decorative elements on monuments and churches, on bees' hives or on certain articles of clothing. Once again, it was only when the mathematicians began to take an interest in the subject - from the middle of the 19th century - that accurate definitions and classifications were established, resulting in a theory. However, the intuitive definition is enough to grasp what the "tiling system" concept entails: a surface type set by assembling basic parts without blank spaces and without overlapping, using a minimal number of different constituent parts (occasionally just one - think of the tiles in our bathrooms or the honeycombs of bees' nests).
This fundamental principle is nonetheless highly elaborate, especially when several types of component parts are combined and when they are coloured in a a given order. This can produce results that are both visually pleasing and fascinating. This is why from the dawn of time until the present day, and in all civilisations, craftsmen and decorators have made extensive use of these effects. Arab artists, in particular, achieved supreme mastery in this art, only to be equalled in our modern age by the famous engraver Escher. As Islam forbids the depiction of animate objects, the Arab artists and craftsmen turned their hand to producing varied and attractive decorative objects through the sole medium of geometry.
While such effects are visually pleasing, they obey precise mathematical laws, even when they were applied before they were discovered ! This was a field in which practice took a lead of several centuries over theory. True, the Greek scholars had already established a few simple rules on the subject. More importantly, the Greek scholars grasped certain key principles such as the answer to the question of the discrete or continuous structure of matter, which triggered a famous dispute between Plato et Aristotle known as the "atomism quarrel", involving considerations on the filling of space with regular shapes, extended to the tiling of a given area. These questions were barely touched on again before the modern age, except by a few pioneers such as the great astronomer Kepler who devoted long and fruitful research to it at the beginning of the 17th century. However, the findings of such research were completely forgotten for three hundred years ! These early discoverers included Father Truchet, as we have already mentioned.
Only at the end of the 19th century, with the growth of crystallography, did the mathematicians take an interest in these issues by applying the theory of groups founded a few decades earlier by Gallois. A major fact that became increasingly apparent at the beginning of the 20th century is that (sic) "the geometry of tiling systems underlies all atomic structures". Throughout the present century, crystallographers and mathematicians, by a kind of process of cross-fertilisation, advanced the theory of tiling systems. In the mid 1980s, this theory provided the key to understanding the existence of quasi crystals hitherto thought to be either non existent or inconceivable.
However, in this rich and complex field many questions remain pending, some of which may be worded in a single line. Nevertheless, the findings of the tiling theory are used today in a range of different fields: computer science, image processing, micro typography, static physics, biology, mesh processing for digital modelling.
Here too father Truchet was a leading, although neglected, forerunner. So let us return to him or rather to Fournier: when Truchet was commissioned to work on the Park of Versailles project, his work was continued a few years later by another monk, the Dominican Douat who published a book (Méthode pour faire une infinité de desseins différents...) that Fournier read ten years later.
Fournier, formerly a wood engraver, developed an interest in ornaments which had hitherto been engraved mainly on wood. The contribution of Fournier was three-fold:
Apart from a few pages in his Manuel, Fournier seems to have made scant use of these ornaments to produce "tiles". in the other hand, Truchet's principle (squarre tiles divided into two colours by a diagonal line) are present in Luce's ornaments and Fournier's Mauel (One third of these 165 ornaments exhibit this symetry), as well as in Bodoni, Caslon, Peignot, etc.'s work.
If some tarotees are set with types (instrad of engraving techniques, such as the ones by Jean-François Rosart, 1768), they do not are real Truchet's tiles. However, modern tarotees (engraved on cupper plates) are real Truchet's tiles, like the ones on the following figures and from Laurent and Deberny's or Peignot's specimens.
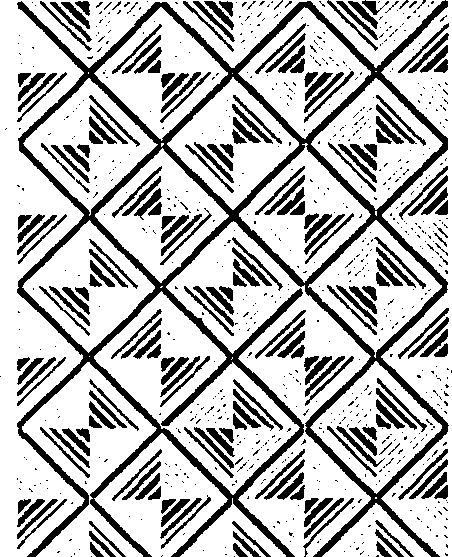
Tarot based on a Truchet tile (symmetry D) - Fondry Schelter & Giesecke, Leipzig, 1876.
Today, fonts offer woodornaments and fleurons that do not give full justice to this particular art. However, from a basic ornament it is easy to create a large number of Truchet tiles by "geometric" composition. These many be easily "programmed" through languages like PostScript or pst-fill.
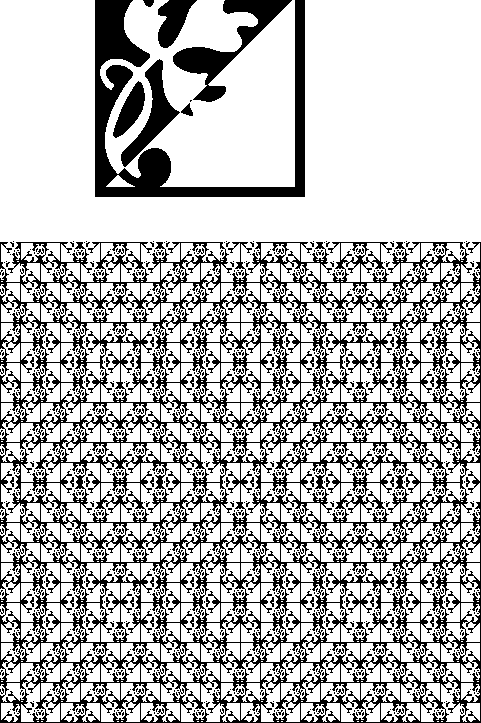
Bottom: a Truchet tiling system (n° 64) executed with (at the top) modern ornamentation
(Adobe Wood Ornament).
Background reading:
Jacques André, formaly
Irisa/Inria-Rennes
Denis Girou,
CNRS,Orsay
Octobre 1998.